Decimal numbers, in my opinion, are harder to visualize than fractions. I mean, the kids have been dealing with fractions, at least in the kitchen, since they were toddlers, but never have I said to them anything like "this recipe calls for .25 cups of sugar." Maybe I should have.
It's even more important, then, that the kids have access to decimal manipulatives when learning these concepts. Unfortunately, Will loathes manipulatives (she knows that solving a problem with manipulatives takes waaaay longer than using an algorithm, and always wants to just skip to the shortest method possible), and so I let her get a couple of lessons into decimals before I pulled them out for her, specifically when I saw that she was having trouble reading the difference between tenths and hundredths in a decimal number.
I mean, if you're going to mistake a tenth for a hundredth, and a hundredth for a tenth, then you REALLY don't understand what that decimal number represents.
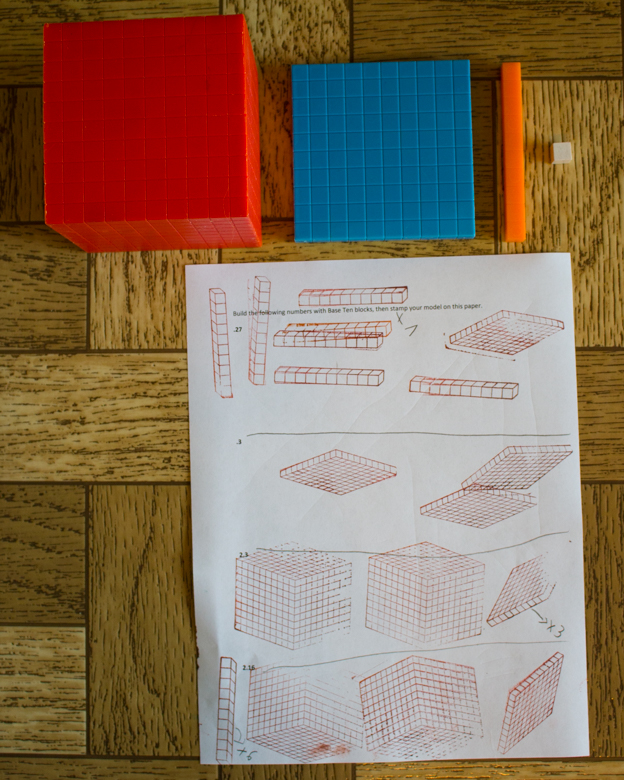
Fortunately, decimals ARE easy to represent, using the very same Base Ten blocks that Will has been using since she was three years old:
Above, you see a representation of the decimal system, as well as a sheet of models that I asked Will to make as part of her math one day.
The way that I introduced Base Ten blocks as decimals to Will may be different from the way that you'd need to introduce them to your kiddos--Will is perfectly comfortable with Base Ten blocks, and had a few lessons on decimals prior to this, and so understood the concept of tenths, hundredths, and thousandths.
To begin, then, I handed her a thousand cube and told her, "Imagine that instead of representing one thousand, this thousand cube now represents one whole. Now, if the thousand represents one whole, what block represents tenths?"
Since Will is familiar with Base Ten blocks, she knows that ten hundred flats make one thousand, and since she's had a couple of lessons on decimals, she knows what a tenth is, so she thought for several seconds, then labeled the hundred flat as the representation of a tenth. To reinforce, we used the hundred flats to count up to one whole.
The next step is to ask, "If this cube represents one whole, what block represents the hundredth?"
This one confused Will, probably because she's so familiar with the idea of one hundred unit cubes, so she first picked out the unit, and we talked about that for a while, and I had her lay out ten bars to cover the hundred flat, and asked her how many of those ten bars would equal one thousand cube. A hundred? Well, then the ten bar must represent the hundredth.
After that, the unit cube was easy to pick out as the thousandth.
I then gave Will several decimal numbers and asked her to model them using the Base Ten stamps (I'd rather have had her build them with the blocks, but she'd have flat-out rebelled at that). I should have given her more room to work, but I thought that she was clever with using the space that she had--for instance, a hundred flat stamp with a "x3" written next to it means "three tenths."
Another absolutely essential manipulative for dealing with decimals is a number line that is marked with hundredths and thousandths. I had to Google Image for a while before I found one that I liked, but when I did, I laminated it so that it can be re-used and the kids can write on it with dry-erase markers. It really only has to go up to maybe 5 or so, as it's just another method of having the kids model the numbers, and so you can simply only have them model numbers between 0 and 5. I call this essential because it's another way to visualize the extreme difference between a tenth and a hundredth and a thousandth. With a number line, there's no WAY that you can mistake 2.4 as 2.04, something that's so easy to do if you have no concept of those numbers in your head.
A number line like this is also essential for demonstrating the concept of rounding numbers, with decimals or without. Will was having a lot of trouble with rounding down--she kept wanting to round 7.1, say, down to 6--until I asked her to mark each number on the number line. Since the goal of rounding is to go to the closest integer, it's perfectly clear, when you look at a number on a number line, where it should go. And of course, you explain the concept of 5 rounding up as mere convention.
Syd is super into fractions right now, so I'm planning an extra unit on them for next week, and it'll be simple to also make that a decimal unit. Pizza will of course be involved, as will some of the other activities from my Homeschool: Math: Fractions and Decimals pinboard, but if you've got some other suggestions for fraction and decimal enrichment, definitely let me know!
No comments:
Post a Comment